how to find horizontal asymptotes

Written By darrelljohnnieriggs Tuesday, February 1, 2022 Add Comment Edit

Below are the points to remember to find the horizontal asymptotes. 3 approaches as x increases y 3x 3 is a slant asymptote leading coefficien t of Dx.

3 6 Finding Horizontal Asymptotes Math Showme

Read the next lesson to find horizontal asymptotes.

. A horizontal asymptote y b exists if the limit of the function equals b as x approaches infinity from both the right and left sides of the graph. Since the x 2 x2 x 2 terms now can cancel we are left with 3 4 frac34 4 3 which is in fact where the horizontal asymptote of the rational function is. If the quotient is constant then y this constant is the equation of a horizontal asymptote.

It can be expressed by y a where a is some constant. The horizontal asymptote of a function f x is a straight parallel line to the x-axis that the function f x approaches as it approaches infinity as we mentioned before. A horizontal asymptote is simply a straight horizontal line on the graph.

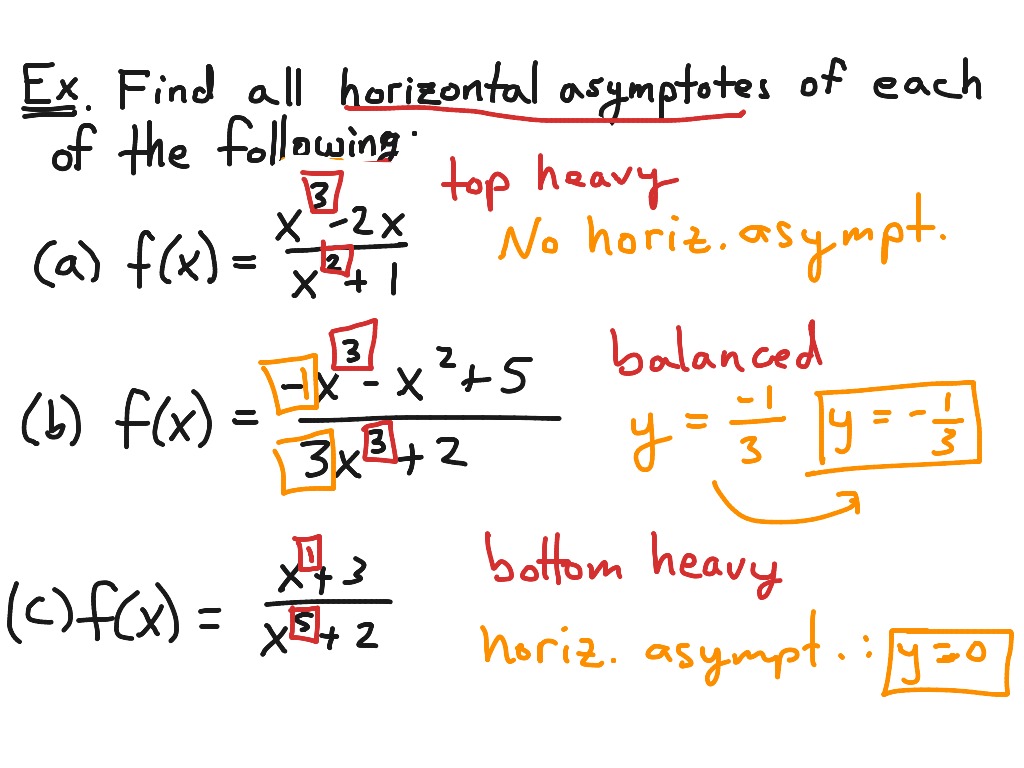
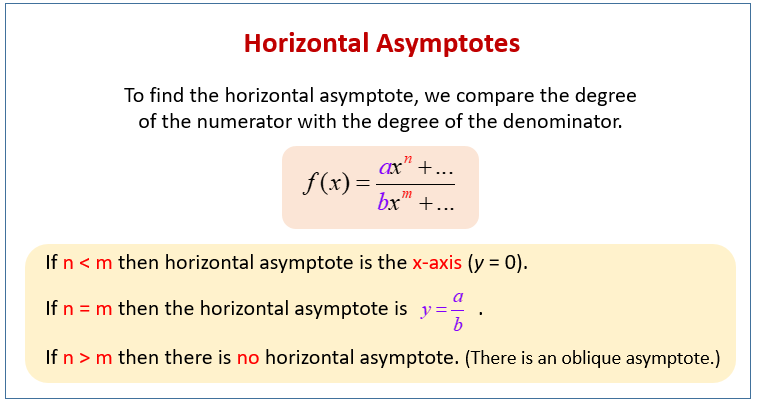
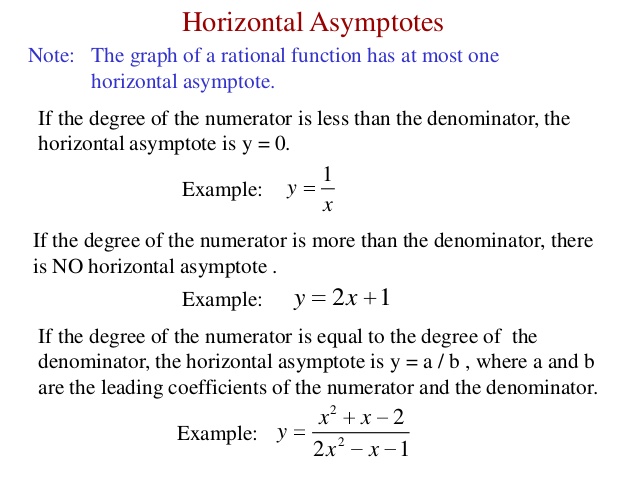
For the rational function fx If the degree of x in the numerator is less than the degree of x in the denominator then y 0 is the horizontal asymptote. If both polynomials are the same degree divide the coefficients of the highest degree terms. If the degree of the polynomial in the numerator is equal to the degree of the polynomial in the denominator we divide the coefficients of the terms with the largest degree to obtain the horizontal asymptotes.

Our horizontal asymptote guidelines are primarily based totally on those stages. What Are Horizontal Asymptotes and How Do You Find Them. Y numerators leading coefficient denominators leading coefficient.

The calculator can find horizontal vertical and slant asymptotes. As you can see the degree of numerator is less than the denominator hence horizontal asymptote is at y 0 Fun Facts About Asymptotes 1. Finding Horizontal Asymptotes of Rational Functions.

Examine how the functions graph approaches the ends of the graph and grows closer and closer to that line. If the degree of the numerator is equal to the degree of the denominator then the horizontal asymptote is the ratio of the leading coefficients of the polynomial functions. The asymptote calculator takes a function and calculates all asymptotes and also graphs the function.

When n is much less than m the horizontal asymptote is y zero or the x-axis. 2 Find horizontal asymptote for fx x x 2 3. In a case like 3 x 4 x 3 3 4 x 2 frac3x4x3 frac34x2 4 x 3 3 x 4 x 2 3 where there is only an x x x term left in the denominator after the reduction process above the horizontal asymptote is at 0.

It can be expressed by y a where a is some constant. Another way of finding a horizontal asymptote of a rational function. Hopefully you can see that an asymptote can often be found by factoring a function to create a simple expression in the denominator.

As x goes to negative or positive infinity the value of the function approaches a. If the degree of the polynomials both in numerator and denominator is equal then divide the coefficients of highest. Select the correct choice below and if necessary fill in the answer boxes to complete your choice.

Divide Nx by Dx. How to determine the horizontal Asymptote. The upper horizontal asymptote is and the lower horizontal asymptote is.

Enter the function you want to find the asymptotes for into the editor. 3x 5x 5 fx 5x 2x-2 4 Find the horizontal asymptotes. Identify horizontal asymptotes Solution.

Find the equation for any horizontal asymptotes for the function below. Because the degrees are equal there will be a. The function has.

Because because approaches 0 as x increases. As a result our function is a product of two polynomials. To find horizontal asymptotes of a function y fx we use the formulas y lim ₓ fx and y lim ₓ -.

If the degree of the numerator is less than the degree of the denominator then the horizontal asymptotes will. That denominator will reveal your asymptotes. Approaches 0 as x increases.

These are known as rational expressions. If the degree of the numerator is less than the degree of the denominator then the horizontal asymptote is equal to the x-axis or y 0. That vertical line is the vertical asymptote x-3.

The horizontal asymptote formula can thus be written as follows. If the polynomial in the numerator is a lower degree than the denominator the x-axis y 0 is the horizontal asymptote. By Free Math Help and Mr.

How to Find Horizontal Asymptotes Using Limits. To find the horizontal asymptotes we have to remember the following. When n is more than m there may be no horizontal asymptote.

Find the horizontal asymptote and interpret it in context of the problem. Both the numerator and denominator are linear degree 1. Y y0 where y0 is a fixed number of finite values.

Y 0 is our horizontal asymptote. Solution fx x x 2 3. If the degree of the denominator is greater than the degree of the numerator horizontal asymptote is at y 0.

If any of these limits results in a non-real number then just ignore that limit. This is always true. When the degrees of the numerator and the denominator are the same then the horizontal asymptote is found by dividing the leading terms so the asymptote is given by.

Also when n is same to m then the horizontal asymptote is same to y ab. The line y L is called a horizontal asymptote of the curve y fx if either. Use the definition of Horizontal Asymptote.

Lets take a look at one to see what a horizontal asymptote looks like.

How To Find Vertical Asymptotes Kristakingmath Youtube